Welcome to the web edition of Marcel Tolkowsky's Diamond Design, as edited by Jasper Paulsen. The original edition was published in 1919 by E. F. N. Spon, Ltd. This web edition is copyright 2001 by Jasper Paulsen.

In 1919, Marcel Tolkowsky published Diamond Design. He systematically analyzed the optics of a diamond. He estimated the best proportions for cutting round brilliant diamonds. With minor changes, today's standards for "ideal cut" diamonds are based on Tolkowsky's book.

This web edition contains the complete, unabridged text of Tolkowsky's landmark book, complete with all of the original illustrations. While editing Tolkowsky's book, I found several errors. Most of the errors were minor math errors. I kept all of Tolkowsky's math errors, but added editor's notes that correct the math errors.

Unfortunately, Tolkowsky's Diamond Design has an even bigger error:  Diamond Design uses a knife-edge girdle. Knife-edge girdles are fragile. As shown in figure 1, modern diamonds leave a layer of material at the girdle, to prevent breakage. This girdle layer changes the optics of the diamond slightly. I have written a separate article, "Diamond Girdles -- How Adding a Girdle Changes Marcel Tolkowsky's Diamond Design."

All three sets of results -- Tolkowsky's values, the editor's notes formulas, and how the girdle changes the formulas -- are shown in Figure 3 below.

If you are beginning to learn about diamonds, you may want to learn more before reading this book. Figure 1 shows the proportions of a diamond. Figure 2 shows the names of the facets of a diamond.
(The facet shapes are from Diamond Design.
 Modern diamonds have longer star and lower girdle facets.)
PriceScope's diamond tutorial explains crown angles, pavilion angles, and table sizes. It talks about good things (like brilliance, fire, and scintillation) and bad things (like knife-edge girdles, too-thick girdles, fish-eyes, and nail-heads):
http://www.pricescope.com/tutorial.asp

Tolkowsky focused on diamonds with a pavilion angle of 40° 45'. He wrote that this angle "gives the most vivid fire and the greatest brilliancy, and that although a greater angle would give better reflection, this would not compensate for the loss due to the corresponding reduction in dispersion."

Tolkowsky also developed a geometric model of the crown, on pages 80-94 of Diamond Design. To keep his model simple, Tolkowsky used a knife-edge girdle. This geometric model let him take a given pavilion angle and calculate the best crown angle and table size for that pavilion angle. Tolkowsky gave only one example: He calculated that a diamond with a pavilion angle of 40° 45' looks best with a crown angle of 34° 30', and a table size of 53%. This example includes some rounding errors. This point is starred (*) in Figure 3.

Tolkowsky's geometric model of the crown can be used to calculate the crown angles and table sizes that correspond to other pavilion angles. But it is hard to draw geometric models very accurately. Even fig. 35 in Diamond Design has inaccuracies.

To get around this problem, the editor's notes convert Tolkowsky's geometric model of the crown into formulas. Tolkowsky's assumptions about the crown (including the knife-edge girdle) have been kept, and his logic has been followed step-by-step. The editor's notes do NOT have his rounding errors, however. The bold line in Figure 3 shows points that satisfy these formulas.

As Tolkowsky writes,

"The calculations have been made as simple as possible, so as not to be beyond the range of readers with a knowledge of elementary geometry, algebra, and trigonometry.  Where, however, it was found that the accuracy of the results would be impaired without the introduction of more advanced mathematics, these have been used, and graphical methods have been explained as an alternative."

Despite being so simple, the conclusions are surprisingly accurate. Figure 3 broadly agrees with actual diamonds, and with 3-D computerized simulations of diamonds. The simulations agree that a larger pavilion angle can be offset by a smaller crown angle. (The simulations suggest slightly smaller pavilion angles. The difference is about the same as Tolkowsky's rounding error.)

Tolkowsky writes that "the high-class brilliant is cut as near the theoretic values as is possible in practice, and gives a magnificent brilliancy to the diamond."

Figure 3 compares three sets of results:

1. Tolkowsky used a geometric model to find the best crown angle and table ratio for one pavilion angle. After rounding errors, he came up with:
   • a pavilion angle of 40° 45'
   • a crown angle of 34° 30'
   • a table ratio of 53%.
     (The table ratio is the largest diameter of the table divided by the average diameter of the diamond.)
   This point is starred (*) in figure 3.
2. My editor's notes to Diamond Design correct Tolkowsky's rounding errors, and show how the best crown angle and table ratio depend on the pavilion angle. These results are shown by the bold curves in figure 3. Unfortunately, the bold curves still use a knife-edge girdle.
3. My girdle article shows how adding a girdle changes Tolkowsky's Diamond Design.
   • The thin curves in figure 3 show diamonds whose thin parts of the girdle are 0.0 % of their diameter (at the "scallops"), and whose thick parts of the girdle are 1.7 % of their diameter (at the "mains"). These diamonds are fragile, because of their thin girdles.
   • The long-dashed curves in figure 3 show diamonds whose thin parts of the girdle are 1.0 % of their diameter (at the "scallops"), and whose thick parts of the girdle are 2.7 % of their diameter (at the "mains"). These girdles are nearly ideal for large diamonds. (They are "medium" for 6.0 mm to 8.0 mm diameters, and "slightly thick" above 8.0 mm diameters.)
   • The short-dashed curves in figure 3 show diamonds whose thin parts of the girdle are 2.0 % of their diameter (at the "scallops"), and whose thick parts of the girdle are 3.7 % of their diameter (at the "mains"). These girdles are "thick" for large diamonds, but are nearly ideal for medium-sized diamonds. (They are "medium" for 4.3 mm to 5.7 mm diameters, and "slightly thick" for 5.7 mm to 6.6 mm diameters.)
     
     To keep a constant total girdle thickness (at the thick parts = "mains"), small diamonds need a larger percentage girdle thickness. Martin Haske has posted more information about diamond girdles at: http://www.gis.net/~adamas/cut.html#girdle

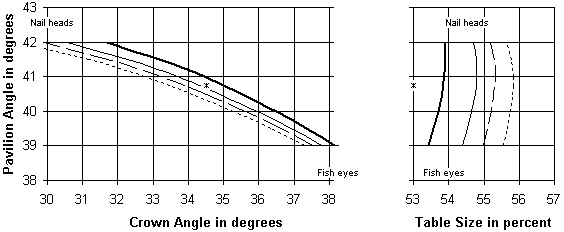
Figure 3 is taken from my article on diamond girdles. Some conclusions:

1. The differences between the stars (*) and the bold curves are due to Tolkowsky's rounding errors. Tolkowsky's rounding error on the crown angle partly offsets his error from using a knife-edge girdle. Tolkowsky's rounding error on the table size seems to go in the wrong direction.
2. The thin curves and the dashed curves show "How Adding a Girdle Changes Marcel Tolkowsky's Diamond Design." My model assumes that the thickness of the "girdle" and "Girdle Wave" reduces the brilliance of the diamond. To partly offset this, the crown is made thinner, both by reducing the crown angle and increasing the table size.
3. Figure 3 broadly agrees with actual diamonds, and with 3-D computer simulations by Moscow State University, the Gemological Institute of America, and Garry Holloway. The simulations agree that a larger pavilion angle can be offset by a smaller crown angle. (The simulations suggest slightly different pavilion angles. The differences are similar to Tolkowsky's rounding error.)
4. Caution: The model breaks down at both ends of the range.
   Editor's notes 30-34 shows that some diamonds that match the formulas are NOT ideal.
   • Extrapolating to smaller pavilion angles makes fish-eyes.
   • Extrapolating to larger pavilion angles makes nail-heads.

If you have evidence that any part of any curve on this graph describes poor diamonds, please let me know.

FOLDS.NET has software for calculating the proportions of Tolkowsky's diamonds.

• "Diamond Cross-Section" lets you adjust Tolkowsky's geometric model. It uses the formulas in the editor's notes of Diamond Design and the girdle article. You can choose the pavilion angle (between 39 and 42 degrees). You can choose either a knife-edge girdle (a la Tolkowsky) or a real girdle (between 0 and 3 percent at the thin parts). (If you want to try unusual values, please use a different program, like CrossSection or GirdledSection .)
  
  You can choose to see just the results, or see the step-by-step calculations. Given your parameters, the program draws a custom version of Tolkowsky's Figure 35. The program calculates the crown angle and table ratio that correspond to your pavilion angle angle and girdle thickness.


• CrossSection.class is the Java 2 program that calculated the bold curves in Figure 3. CrossSection.class is part of "Diamond Cross-Section".


• GirdledSection.class is the Java 2 program that calculated the thin curves and dashed curves in Figure 3. GirdledSection.class is part of "Diamond Cross-Section".


• DiamondDimension.exe is a Win32 console application that calculates the lengths of all of the edges of a perfectly symmetrical diamond. DiamondDimension.exe makes some assumptions (about the star facets, upper girdle facets, and lower girdle facets) that are different from Tolkowsky's recommendations.

If you want to install or use CrossSection.class, GirdledSection.class, or DiamondDimension.exe, please visit http://www.folds.net/diamond/software_help.html.

The software is copyright 2001 by Jasper Paulsen. All rights reserved. Some of the code is taken from sources listed at http://www.folds.net/diamond/software_help.html. Your comments and questions are welcomed.

Acknowledgements: I thank the late Marcel Tolkowsky for writing Diamond Design. I thank everyone kind enough to criticize this web-site, and tell me things that I should fix. Many people (listed on the software help page) have provided programming suggestions and/or code snippets. Garry Holloway, Marvin Leib, Bruce Harding, Martin Haske, Jefferson Lewis, and Iiro Suokko have pointed out errors and omissions. If you notice one, please let me know, and I will do my best to fix it promptly.

DISCLAIMER: This information and software are for educational purposes only and should not be used in place of services from a professional appraiser. Formulas cannot be 100% accurate due to subtleties not included in the formulas. All decisions should be based on your own knowledge. I cannot accept any responsibility for decisions you make.

Thank you for visiting FOLDS.NET.
The first edition was published in 1919 by Marcel Tolkowsky in the United States and the United Kingdom.
Web formatting, transcription, figures 38 and 39, editor's notes, and this page are copyright 2001 by Jasper Paulsen.
Your comments are welcomed.
Last updated January 4, 2002.